Types of Production Functions: Cobb Douglas, Leontief, CES

Types of Production Functions in Economics

Table of Content [Show]

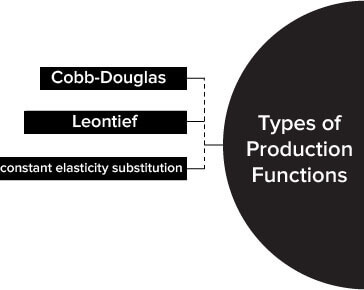
There are different types of production functions that can be classified according to the degree of substitution of one input by the other.

Figure illustrates different types of production functions:

Cobb Douglas Production Function

The Cobb Douglas production function, given by American economists, Charles W. Cobb and Paul. H Douglas, studies the relation between the input and the output.

The cobb douglas production function is that type of production function wherein an input can be substituted by others to a limited extent.

For example, capital and labour can be used as a substitute of each other, however to a limited extent only. Cobb Douglas production function can be expressed as follows:

Q = AK^a L^b

Where,

A = positive constant
a and b = positive fractions
b = 1–a

Another way of expressing Cobb- Douglas production function is:

Q = AK^a L^1-a

Let us take up an example to understand the calculations involved in the cobb-Douglas function.

Assume A = 40, a = 0.3 and b= 0.7 , K = 3 and L = 5

Therefore,

Q = 40 (3)0.3 (5)0.7
log Q = log 40 + 0.3 log 3 + 0.7 log 5
log Q = 1.6020 + (0.3 X 0.4771) + (0.7 X 0.6989)
log Q = 1.6020 + 0.1431 + 0.4892
log Q = 2.2343

Now, we will take the antilog of the above to get the value of Q. antilog log Q = antilog 2.2343

Q = 171.5141

Cobb Douglas Production Function Properties

The main attributes of the Cobb Douglas production function are discussed as follows:

It enables the conversion of the algebraic form into log linear form, represented as follows:
log Q = log A + a log K + b log L

This production function has been estimated with the help of linear regression analysis.
It acts as a homogeneous production function, whose degree can be calculated by the value obtained after adding values of a and b. If the resultant value of a+b is 1, it implies that the degree of homogeneity is 1 and indicates the constant returns to scale.
It makes use of parameters a and b, which signifies the elasticity coefficients of output for inputs, labour and capital, respectively. Output elasticity coefficient is the change in output that occurs due to adjustment in capital while keeping labour at constant.
It depicts the non-existence of production at zero cost.

Leontief Production Function

Leontief production function, evolved by W. Wassily Leontif, uses fixed proportion of inputs having no substitutability between them.

It implies that if the input-output ratio is independent of the scale of production, there is existence of Leontief production function. It assumes strict complementarity of factors of production. Leontief production function is also called as fixed proportion production function.

This production function can be expressed as follows:

q= min (z₁/a, z₂/b)

where, q = quantity of output produced
z₁ = utilised quantity of input 1
z₂ = utilised quantity of input 2
a and b = constants

Minimum implies that the total output depends upon the smaller of the two ratios.

The coefficients a and b are the fixed input requirements for producing a single unit of output. It means that if we want to produce q units of output, we need aq units of capital (z₁) and bq units of labour (z₂).

Or we can mathematically state that, z₁ = aq represents the capital requirements and z₂ = aq represents the labour requirements.

Therefore, z₁ / z₁ = a/b. that is, there is a particular fixed proportion of capital and labour required to produce output. That is if we increase one of the factors without increasing the other factor proportionally, then there will be no increase in output.

For example, suppose the Leontief production functions for goods X is

X = min [ 3 Lx , 7 Kx]

This implies that for producing a single unit of X, a minimum of 1/3 unit of labour and 1/7 unit of capital would be required.

X = min [ Lx / (1/3) , Kx / (1/7)]

In this case, the values 1/3 and 1/7 depict labour activities and therefore, are activity coefficients as they depict labour activities.

Now, say 1/3 of a day’s work is required to produce 1 unit of X, and the activity required of capital 1/7 of a day’s work to produce 1 unit of X.

With Lx = 5 and Kx = 3, X = min [ 15, 21] = 15 units of X.

Therefore, with 15 units of X ; Lx = 5 and Kx = 2, you get X = min [15, 14] = 14 units of X.

The logical capital-labor ratio in the X industry to choose will be Kx / Lx = 3/5 = 0.6.

CES Production Function

CES stands for constant elasticity substitution. CES production function displays a constant change produced in the output due to change in input of production.

It is expressed as:

Q = A [aK^–β + (1–a) L^–β]^–1/β

CES has the homogeneity degree of 1 that implies that output would be increased with the increase in inputs. For example, labour and capital has increased by constant factor m.

Q’ = A [a (mK)–β + (1–a) (mL)–β]–1/β
Q’ = A [m–β {aK–β + (1–a) L– β}]–1/β
Q’ = (m–β) –1/β .A [aK–β + (1-a) L–β]-1/β
Because, Q = A [aK–β + (1-a) L–β]-1/β

Therefore, Q’ = mQ

This implies that CES production function is homogeneous with degree one.

For example, let us assume that the CES production function’s parameters are as follows:

A = 1.0
a = 0.3
b = 0.7
β = 0.18
s = 1/(1+β) = 1/(1+0.18) = 0.847
L = 50
K = 30

The CES production function will be formed as follows:
Q = A [aK–β + (1–a) L–β]–1/β
Q = 1.0 * .3 X (L0.18) + .7 X (K0.18)
Q = 1.0 * .3 X 500.18 + .7 X 300.18
Q = 1.0 * .3 X 1 + .7 X 1
Q = 1.0 * 1
Q = 1.0 / 11/.18
Q = 1

Properties of CES Production Function

The properties of CES function are as follows:

The value of elasticity of substitution depends upon the value of a.
The marginal products are positive and slope downwards.

Merits of CES Production Function

The merits of CES production are as follows:

Covers a number of parameters, such as efficiency and substitutability
Easy to estimate
Free from unrealistic assumptions, such as fixed technology, etc.

Demerits of CES function

The demerits of CES production system are as follows:

Fails to fit for manufacturing industries
Cannot be generalised in case of n factors of production
Fails to give correct economic implications