Now that we know how to locate the center of mass of a system of particles, we discuss how external forces can move a center of mass. Let us start with a simple system of two billiard balls.
If you roll a cue ball at a second billiard ball that is at rest, you expect that the two-ball system will continue to have some forward motion after impact. You would be surprised, for example, if both balls came back toward you or if both moved to the right or to the left.
What continues to move forward, its steady motion completely unaffected by the collision, is the center of mass of the two-ball system. If you focus on this point—which is always halfway between these bodies because they have identical masses—you can easily convince yourself by trial at a billiard table that this is so. No matter whether the collision is glancing, head-on, or somewhere in between, the center of mass continues to move forward, as if the collision had never occurred. Let us look into this center-of-mass motion in more detail.
To do so, we replace the pair of billiard balls with an assemblage of n particles of (possibly) different masses. We are interested not in the individual motions of these particles but only in the motion of the center of mass of the assemblage. Although the center of mass is just a point, it moves like a particle whose mass is equal to the total mass of the system; we can assign a position, a velocity, and an acceleration to it. We state (and shall prove next) that the vector equation that governs the motion of the center of mass of such a system of particles is

This equation is Newton’s second law for the motion of the center of mass of a system of particles. Note that its form is the same as the form of the equation ![]() for the motion of a single particle. However, the three quantities that appear in Eq. 9-14 must be evaluated with some care:
for the motion of a single particle. However, the three quantities that appear in Eq. 9-14 must be evaluated with some care:
1. ![]() is the net force of all external forces that act on the system. Forces on one part of the system from another part of the system (internal forces) are not included in Eq. 9-14.
is the net force of all external forces that act on the system. Forces on one part of the system from another part of the system (internal forces) are not included in Eq. 9-14.
2. M is the total mass of the system. We assume that no mass enters or leaves the system as it moves, so that M remains constant. The system is said to be closed.
3. ![]() is the acceleration of the center of mass of the system. Equation 9-14 gives no information about the acceleration of any other point of the system.
is the acceleration of the center of mass of the system. Equation 9-14 gives no information about the acceleration of any other point of the system.
Equation 9-14 is equivalent to three equations involving the components of ![]() and
and ![]() along the three coordinate axes. These equations are
along the three coordinate axes. These equations are
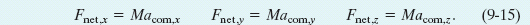
Now we can go back and examine the behavior of the billiard balls. Once the cue ball has begun to roll, no net external force acts on the (two-ball) system. Thus, because ![]() , Eq. 9-14 tells us that
, Eq. 9-14 tells us that ![]() also. Because acceleration is the rate of change of velocity, we conclude that the velocity of the center of mass of the system of two balls does not change. When the two balls collide, the forces that come into play are internal forces, on one ball from the other. Such forces do not contribute to the net force
also. Because acceleration is the rate of change of velocity, we conclude that the velocity of the center of mass of the system of two balls does not change. When the two balls collide, the forces that come into play are internal forces, on one ball from the other. Such forces do not contribute to the net force ![]() , which remains zero. Thus, the center of mass of the system, which was moving forward before the collision, must continue to move forward after the collision, with the same speed and in the same direction.
, which remains zero. Thus, the center of mass of the system, which was moving forward before the collision, must continue to move forward after the collision, with the same speed and in the same direction.
Equation 9-14 applies not only to a system of particles but also to a solid body, such as the bat of Fig. 9-1b. In that case, M in Eq. 9-14 is the mass of the bat and ![]() is the gravitational force on the bat. Equation 9-14 then tells us that
is the gravitational force on the bat. Equation 9-14 then tells us that ![]() . In other words, the center of the bat moves as if the bat ware a single particle of mass M, with force
. In other words, the center of the bat moves as if the bat ware a single particle of mass M, with force ![]() acting on it.
acting on it.
Figure 9-5 shows another interesting case. Suppose that at a fireworks display, a rocket is launched on a parabolic path. At a certain point, it explodes into fragments. If the explosion had not occurred, the rocket would have continued along the trajectory shown in the figure. The forces of the explosion are internal to the system (at first the system is just the rocket, and later it is its fragments); that is, they are forces on parts of the system from other parts. If we ignore air drag, the net external force ![]() acting on the system is the gravitational force on the system, regardless of whether the rocket explodes. Thus, from Eq. 9-14, the acceleration
acting on the system is the gravitational force on the system, regardless of whether the rocket explodes. Thus, from Eq. 9-14, the acceleration ![]() of the center of mass of the fragments (while they are in flight) remains equal to
of the center of mass of the fragments (while they are in flight) remains equal to ![]() . This means that the center of mass of the fragments follows the same parabolic trajectory that the rocket would have followed had it not exploded.
. This means that the center of mass of the fragments follows the same parabolic trajectory that the rocket would have followed had it not exploded.
When a ballet dancer leaps across the stage in a grand jeté, she raises her arms and stretches her legs out horizontally as soon as her feet leave the stage (Fig. 9-6). These actions shift her center of mass upward through her body. Although the shifting center of mass faithfully follows a parabolic path across the stage, its movement relative to the body decreases the height that is attained by her head and torso, relative to that of a normal jump. The result is that the head and torso follow a nearly horizontal path, giving an illusion that the dancer is floating.
Proof of Equation 9-14
Now let us prove this important equation. From Eq. 9-8 we have, for a system of n particles,
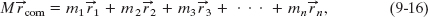

Fig. 9-5 A fireworks rocket explodes in flight. In the absence of air drag, the center of mass of the fragments would continue to follow the original parabolic path, until fragments began to hit the ground.

Fig. 9-6 A grand jeté. (Adapted from The Physics of Dance, by Kenneth Laws, Schirmer Books, 1984.)
in which M is the system’s total mass and ![]() is the vector locating the position of the system’s center of mass.
is the vector locating the position of the system’s center of mass.
Differentiating Eq. 9-16 with respect to time gives
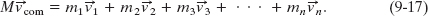
Here ![]() is the velocity of the ith particle, and
is the velocity of the ith particle, and ![]() is the Velocity of the Center of Mass.
is the Velocity of the Center of Mass.
Differentiating Eq. 9-17 with respect to time leads to
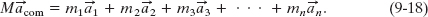
Here ![]() is the acceleration of the ith particle, and
is the acceleration of the ith particle, and ![]() is the acceleration of the center of mass. Although the center of mass is just a geometrical point, it has a position, a velocity, and an acceleration, as if it were a particle.
is the acceleration of the center of mass. Although the center of mass is just a geometrical point, it has a position, a velocity, and an acceleration, as if it were a particle.
From Newton’s second law, ![]() is equal to the resultant force
is equal to the resultant force ![]() that acts on the ith particle. Thus, we can rewrite Eq. 9-18 as
that acts on the ith particle. Thus, we can rewrite Eq. 9-18 as

Among the forces that contribute to the right side of Eq. 9-19 will be forces that the particles of the system exert on each other (internal forces) and forces exerted on the particles from outside the system (external forces). By Newton’s third law, the internal forces form third-law force pairs and cancel out in the sum that appears on the right side of Eq. 9-19. What remains is the vector sum of all the external forces that act on the system. Equation 9-19 then reduces to Eq. 9-14, the relation that we set out to prove.
![]() CHECKPOINT 2 Two skaters on frictionless ice hold opposite ends of a pole of negligible mass. An axis runs along the pole, and the origin of the axis is at the center of mass of the two-skater system. One skater, Fred, weighs twice as much as the other skater, Ethel. Where do the skaters meet if (a) Fred pulls hand over hand along the pole so as to draw himself to Ethel, (b) Ethel pulls hand over hand to draw herself to Fred, and (c) both skaters pull hand over hand?
CHECKPOINT 2 Two skaters on frictionless ice hold opposite ends of a pole of negligible mass. An axis runs along the pole, and the origin of the axis is at the center of mass of the two-skater system. One skater, Fred, weighs twice as much as the other skater, Ethel. Where do the skaters meet if (a) Fred pulls hand over hand along the pole so as to draw himself to Ethel, (b) Ethel pulls hand over hand to draw herself to Fred, and (c) both skaters pull hand over hand?
The three particles in Fig. 9-7a are initially at rest. Each experiences an external force due to bodies outside the three-particle system. The directions are indicated, and the magnitudes are F1 = 6.0 N, F2 = 12 N, and F3 = 14 N. What is the acceleration of the center of mass of the system, and in what direction does it move?
Solution: The position of the center of mass, calculated by the method of Sample Problem 9-1, is marked by a dot in the figure. One Key Idea here is that we can treat the center of mass as if it were a real particle, with a mass equal to the system’s total mass M = 16 kg. We can also treat the three external forces as if they act at the center of mass (Fig. 9-7b).
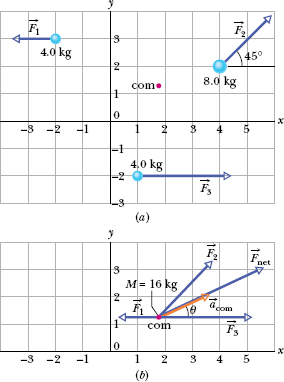
Fig. 9-7 (a) Three particles, initially at rest in the positions shown, are acted on by the external forces shown. The center of mass (com) of the system is marked. (b) The forces are now transferred to the center of mass of the system, which behaves like a particle with a mass M equal to the total mass of the system. The net external force ![]() and the acceleration
and the acceleration ![]() of the center of mass are shown.
of the center of mass are shown.
A second Key Idea is that we can now apply Newton’s second law ![]() to the center of mass, writing
to the center of mass, writing

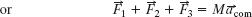
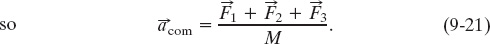
Equation 9-20 tells us that the acceleration ![]() of the center of mass is in the same direction as the net external force
of the center of mass is in the same direction as the net external force ![]() on the system (Fig. 9-7b). Because the particles are initially at rest, the center of mass must also be at rest. As the center of mass then begins to accelerate, it must move off in the common direction of
on the system (Fig. 9-7b). Because the particles are initially at rest, the center of mass must also be at rest. As the center of mass then begins to accelerate, it must move off in the common direction of ![]() and
and ![]() .
.
We can evaluate the right side of Eq. 9-21 directly on a vector-capable calculator, or we can rewrite Eq. 9-21 in component form, find the components of ![]() , and then find
, and then find ![]() . Along the x axis we have
. Along the x axis we have
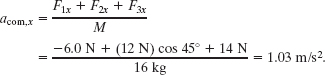
Along the y axis, we have
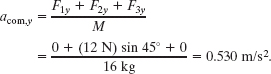
From these components, we find that ![]() has the magnitude
has the magnitude
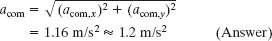
and the angle (from the positive direction of the x axis)

Leave a Reply