A block moving horizontally on a smooth surface with a speed of 40m/s40�/� splits into two equal parts. If one of the parts moves at 60m/s60�/� in the same direction, then the fractional change in the kinetic energy will be x:4�:4 where x� = ___________. (JEE Main 2021)
Sol:
Given:
Initial velocity of the block before splitting into two equal parts, V=40m/s�=40�/�
As the block is split into two equal masses, so if we consider the initial mass of the block m� then after splitting, it will be m2�2. Let the velocity of 1st part be v� and the velocity of the second part according to the question is v′=60m/s�′=60�/�.
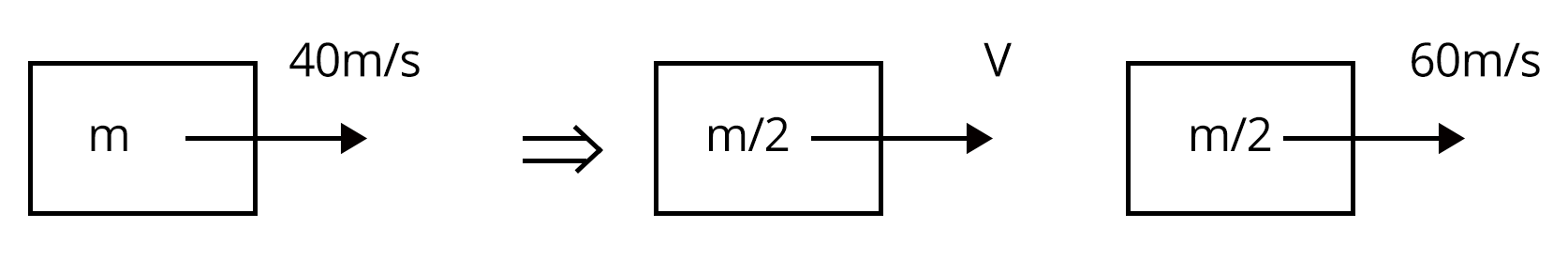
Now, using the momentum conservation principle, we get:
Pi=Pf��=��
mV=m2v+m2v′��=�2�+�2�′
V=v2+v′2=v2+602�=�2+�′2=�2+602
40=v2+3040=�2+30
v=10×2=20m/s�=10×2=20�/�
Now, the initial kinetic energy ((K.E.)i(�.�.)�) is
(K.E.)i=12mV2=12m(40)2=800m(�.�.)�=12��2=12�(40)2=800�
The final kinetic energy ((K.E.)f(�.�.)�) is
(K.E.)f=12m2(20)2+12m2(60)2=1000m(�.�.)�=12�2(20)2+12�2(60)2=1000�
The change in kinetic energy (ΔK.E.Δ�.�.) is
|ΔK.E.|=|1000m−800m|=200m|Δ�.�.|=|1000�−800�|=200�
The fractional change in kinetic energy is
fractional change in K.E.=ΔK.E.(K.E.)i=200m800mfractional change in K.E.=Δ�.�.(�.�.)�=200�800�
fractional change in K.E.=14=x4fractional change in K.E.=14=�4
Hence, the value of x� is 11.
Trick: Just apply the concept of conservation of momentum then fraction change in the kinetic energy.
Leave a Reply