Relative Motion in Two Dimensions

Now we turn from relative motion in one dimension to relative motion in two (and, by extension, three) dimensions. In Fig. 4-21, our two observers are again watching a moving particle P from the origins of reference frames A and B, while B moves at a constant velocity  relative to A. (The corresponding axes of these two frames remain parallel.)

Figure 4-21 shows a certain instant during the motion. At that instant, the position vector of the origin of B relative to the origin of A is . Also, the position vectors of particle P are  relative to the origin of A and  relative to the origin of B. From the arrangement of heads and tails of those three position vectors, we can relate the vectors with

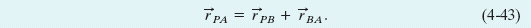
By taking the time derivative of this equation, we can relate the velocities  and  of particle P relative to our observers:

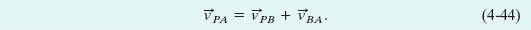
By taking the time derivative of this relation, we can relate the accelerations  and  of the particle P relative to our observers. However, note that because  is constant, its time derivative is zero. Thus, we get

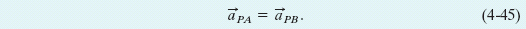
As for one-dimensional motion, we have the following rule: Observers on different frames of reference that move at constant velocity relative to each other will measure the same acceleration for a moving particle.

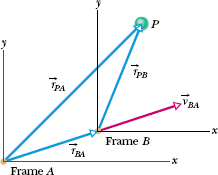
Fig. 4-21   Frame B has the constant two-dimensional velocity  relative to frame A. The position vector of B relative to A is . The position vectors of particle P are  relative to A and  relative to B.

Sample Problem 4-11

In Fig. 4-22a, a plane moves due east while the pilot points the plane somewhat south of east, toward a steady wind that blows to the northeast. The plane has velocity _PW relative to the wind, with an airspeed (speed relative to the wind) of 215 km/h, directed at angle θ south of east. The wind has velocity _WG relative to the ground, with a speed of 65.0 km/h, directed 20.0° east of north. What is the magnitude of the velocity _PG of the plane relative to the ground, and what is θ?

Solution: The Key Idea is that the situation is like the one in Fig. 4-21. Here the moving particle P is the plane, frame A is attached to the ground (call it G), and frame B is “attached” to the wind (call it W). We need to construct a vector diagram like that in Fig. 4-21 but this time using the three velocity vectors.

First construct a sentence that relates the three vectors:

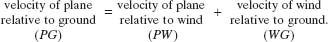
This relation can be drawn as in Fig. 4-22b and written in vector notation as

We want the magnitude of the first vector and the direction of the second vector. With unknowns in two vectors, we cannot solve Eq. 4-46 directly on a vector-capable calculator. Instead, we need to resolve the vectors into components on the coordinate system of Fig. 4-22b and then solve Eq. 4-46 axis by axis (see Section 3-6). For the y components, we find

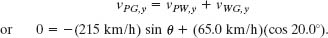
Solving for θ gives us

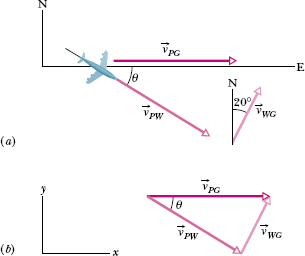
Fig. 4-22   To travel due east, the plane must head somewhat into the wind.

Similarly, for the x components we find

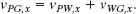
Here, because _PG is parallel to the x axis, the component v_PG,x is equal to the magnitude v_PG. Substituting this notation and the value θ = 16.5°, we find

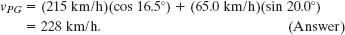
CHECKPOINT 8 Suppose the pilot turns the plane to point it due east without changing the airspeed. Do the following magnitudes increase, decrease, or remain the same: (a) v_PG,y, (b) v_PG,x, (c) v_PG? (You can answer with-out computation.)