The Standard State Gibbs Energy of Reaction

The first term on the right side of Eqns. 17.12 and 17.16, , is called the standard state Gibbs energy of reaction at the temperature of the reaction, which we will denote . The standard state Gibbs energy of reaction is analogous to the standard state heat of reaction introduced in Section 3.6. The standard state Gibbs energy for reaction can be calculated using Gibbs energies of formation.

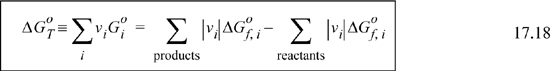
As an example, for CH_4(g) + H₂O_(g) → CO_(g) + 3H_2(g)

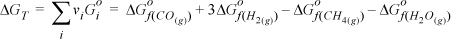
It may be helpful to think of the sum as representing a path via Hess’s law where the reactants are “unformed” to the elements and then “formed” into the products. The signs of the formation Gibbs energies of the products are positive and the signs for the reactants are negative. Thus,

 Standard state Gibbs energy of reaction.

The Gibbs energies of formation are typically tabulated at 298.15 K and 1 bar, and special calculations must be performed to calculate  at other temperatures—the calculations will be covered in Section 17.7. Like the enthalpy of formation, the Gibbs energy of formation is taken as zero for elements that naturally exist as molecules at 298.15 K and 1 bar, and the same cautions about the state of aggregation apply. Gibbs energies of formation are tabulated for many compounds in Appendix E at 298.15 K and 1 bar. Note that for water, the difference between  and  is the Gibbs energy of vaporization at 298 K. The difference is nonzero because liquid is more stable. (Which phase will have a lower Gibbs energy of formation at 298.15 K and 1 bar?)

The standard state Gibbs energy of reaction is related to the equilibrium constant through Eqn. 17.16,

 Relation between Gibbs energy of reaction and the equilibrium constant.

Once the value of the equilibrium constant is known, equilibrium compositions can be determined, as shown in Example 17.1. The next example illustrates calculation of the Gibbs energy of reaction and the equilibrium constant.

---

Example 17.2. Calculation of standard state Gibbs energy of reaction

Butadiene is prepared by the gas phase catalytic dehydrogenation of 1-butene:

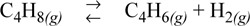
Calculate the standard state Gibbs energy of reaction and the equilibrium constant at 298.15 K.

Solution

We find values tabulated for the standard state enthalpies of formation and standard state Gibbs energy of formation at 298.15 K.

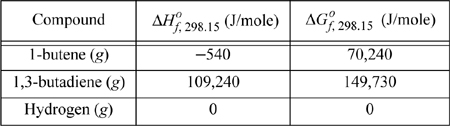
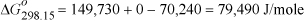
The equilibrium constant is determined from Eqn. 17.16;

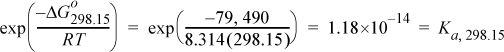
This reaction is not favorable at room temperature because the equilibrium constant is small.

---

Composition and Pressure Independence of K_a

The use of standard states for calculating  has important implications on the composition and pressure independence of K_a. The standard state is at a fixed pressure, P°. Thus,  is independent of pressure. The standard states are also at fixed composition (often pure), and thus  is independent of equilibrium composition. Looking at Eqn. 17.20, we conclude that because  is independent of equilibrium composition and pressure, K_a is independent of equilibrium composition and pressure. One important point is that the state of aggregation in the standard state is important and the values of  and K_a do depend on the state of aggregation in the standard state; this point will be clarified in later sections.

 The equilibrium constant K_a is independent of composition and pressure.

We have now demonstrated steps 2(a), 3, and 4 for the procedure given in Section 17.1. The concepts have been demonstrated, but we must correct the temperature before doing calculations at temperatures other than 298.15 K. The butadiene reaction of Example 17.2 becomes more favorable with a larger K_a at higher temperatures. We will discuss some important aspects of the effects of pressure and inerts and also discuss reaction spontaneity before showing the calculation of temperature corrections.