Precipitates from solid solution

The precipitation of a solid phase from a liquid matrix is governed by a balance between the thermodynamic driving force and the energy penalty for creating new solid-liquid surface interfaces. This determines the size and shape of the precipitates. The precipitation of a solid phase from a solid parent phase is very similar.

There are various types of interface between solid phases:

1. Coherent – there is perfect registry of the lattices.
2. Coherent with strain – it is quite likely for there to be some strain with the interface, due to imperfect matching. The strain energy increases with the size of the growing particle, and there is a transition to a semi-coherent interface.
3. Semi-coherent interface – the introduction of dislocations reduces the strain energy (but they themselves contribute to the energy of the system).
4. Incoherent – there is no matching of the interface.

Coherent	Coherent with strain
Semi-coherent	Incoherent

In general, the interfacial free energy will be minimised with better matching of the two phases. Incoherent interfaces have high energy and are relatively mobile because of the greater freedom of atomic motion.

The stresses present in the parent matrix as the precipitate grows strongly influences the shape of the precipitate. By modelling the precipitate as an ellipsoid of revolution, the following graph shows how the strain energy is related to the shape.

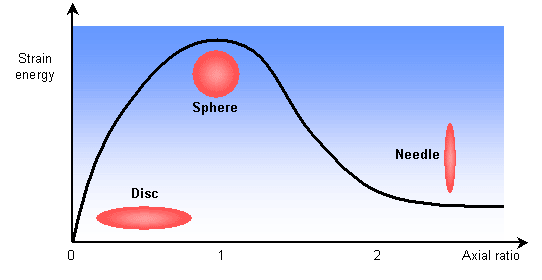
Growth as discs or plates is clearly preferred. A precipitate particle will likely have some coherent and some incoherent interfaces with the matrix. The greater mobility of the incoherent interfaces leads to faster growth in these directions. This anisotropic growth leads to plate and disc morphologies. The bounding coherent interfaces will be parallel to crystallographic planes in the matrix.

Solid solution precipitation/exsolution is used to strengthen many alloys. This is known as precipitation hardening, or age hardening. It involves quenching an alloy to a supersaturated state (where the amount of dissolved solute is greater than the equilibrium amount predicted by the phase diagram). A heating schedule can then be applied to control the nature of the precipitation. For example in Al-Cu, a very fine dispersion of θ particles hardens the α phase. This age-hardened alloy is used in aerospace applications.

View micrograph of a precipitation hardened sample of Al-Cu

At lower temperatures, it is preferable to have incoherent precipitates, as the greater strains produce more resistance on dislocation motion. At higher temperatures however, the greater mobililty of the incoherent interfaces allows larger particles to grow at the expense of smaller ones (called coarsening), and the system becomes less effective at strengthening. So for high temperature use, coherent particles are used, such as the γ’ precipitate in nickel-based superalloys (here a phenomenon called order hardening provides the strengthening mechanism, rather than the strain fields).

View micrograph of an order hardened sample of Nickel superalloy

Ceramics, which are typically brittle, can also benefit from solid solution precipitation. Zirconia based compounds can be toughened by having tetragonal structure particles in the monoclinic matrix. Propagation of cracks through the zirconia requires the transformation of these precipitates to the monoclinic form. This requires an input of energy, provided by the stress, hence the material is toughened.

Another use of solid solution precipitation lies in nano-materials. By precipitating from a solid solution, the nanometre (10^-9 m) scale of the microstructure can provide many beneficial effects. For example, the presence of 5 nm diameter carbide particles in steel piano wires helps make it the world’s strongest structural material (with some ductility). Precipitation from a liquid phase is too fast to produce such small scale particles.